The binding of a proton to an acceptor changes the electrical charge on the acceptor, and this often changes the biochemical properties of the molecule. For example:
When a solution is made up containing 1 mole of solute in 1 litre (1 dm-3) of solvent the concentration of the solute is said to be 1 molar or 1 mol dm-3 (in out_dated notation: 1 M), and this is the standard unit of concentration.
The concentrations of most biochemicals in vivo range between about 10-2 mol dm-3 and 10_12 mol dm-3.
The concentration of protons in a neutral (neither acidic nor basic) aqueous solution is 10_7 mol dm-3.
Manipulating such small numbers is facilitated by using logarithms.
actual number = another numberindex, or exponent
In the days before electronic calculators were readily available to school children (not so long ago!) logarithm and antilogarithm tables were easily available, and we had to have a set handy for maths, physics and chemistry classes and homework.
The following table, in conjunction with the logarithm table, will require little explanation:
| Number | Scientific Notation | Log10 No. |
| 1 | 1 x 100 | 0.0000 |
| 6 | 6 x 100 | 0.7782 |
| 10 | 1 x 101 | 1.0000 |
| 20 | 2 x 101 | 1.3010 |
| 300 | 3 x 102 | 2.4771 |
| 4,000 | 4 x 103 | 3.6021 |
| 5,000,000 | 5 x 106 | 6.6990 |
The log(arithm)10 of the number has two distinct parts separated by a full stop called "point", which is commonly mistaken for, but is not a simple decimal point: the integer to the left of the point is called the characteristic and corresponds to the power to which the multiplier 10 must be raised; the number to the right of the point is called the mantissa and is determined either by calculation or by looking up in the tables . The mantissa is always positive, whereas the characteristic is negative for numbers that are less than 1.0.
The log and antilog tables shown are four figure tables, and these are accurate enough for most applications. For more exacting applications, tables are available with which greater accuracy is possible.
To find the log10 of 45.67 we first inspect the number and determine the value of the characteristic by converting the number to scientific notation : 4.567 x 101. The characteristic is thus 1.
We now look up 4567 in the log tables as follows:
the first two digits are to be found in the first column of the
table. If the number that we were looking for were 4500 the
mantissa would have been: .6532 (always include the point in the
mantissa).
The mantissa for 4560 is .6590, and the one we really want is
.6597 _ having added the number from the appropriate
column in the right hand set of columns to the mantissa of the
first three digits.
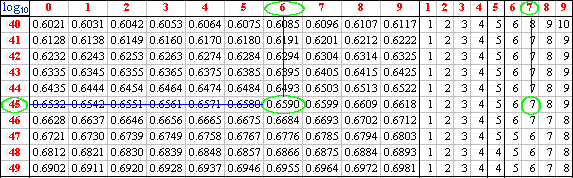
Practice looking up the log10 for a number and type your answer in the box alongside.
The characteristic of the log of this number is called bar three, and the whole log is called:
It is only the characteristic that can be negative; the mantissa is always positive.
This confusion is compounded by the facts that:
Thus log10 0.004545 = -3.6585.
Practice looking up the log10 for a number less than 1 and type your answer in the box alongside.
Now you should be able to reverse the process and use the table to find the number for which the log10 is 2.8388, however it is slightly easier to look up the mantissa in the antilog tables, the characteristic merely tells you where to put the decimal point.
Where a bar characteristic is involved, as with some pH calculations, some understanding is required. For assistance with examples of the latter we recommend that you continue with this document up to the end of the next section on the pH scale; or you can jump immediately to some pH exercises.
These days scientists and their students expecting to have to manipulate logarithms are already equipped with smart phones/tablets with built-in calculators, but here we find another difficulty: not all such devices are easy to use or interpret. We strongly urge that you take time here to practice 'logging' and 'antilogging' numbers with your own calculator application, checking the outputs against the logarithm and tables.
If you are unable to reconcile results, you are advised to:
The aqueous environment of most biochemical reactions can exchange protons with such groups. The proton exchange reaction is governed, as are others, by the concentrations of the reactants, their willingness to participate and the temperature of the system (which is really an indication of the speed of the reactants).
Of the willingness to participate (pK in reactions involving protons), strong acids are more willing to donate their protons than are weak acids: by definition the protons of a strong acid are always fully dissociated in aqueous solutions, whereas the protons of a weak acid show a greater affinity for their conjugate base.
We encourage you to look at the accompanying (free) Windows Titrations program.
Water is both an acid and a base:
H2O + H2O = H3O+ + OH_To what extent does this dissociation of water molecules (protolysis) occur? If we could fill a bucket with pure H2O and allowed it to dissociate (for an infinite length of time) we could then measure the concentrations of the protons or hydroxyl ions present and determine the acid dissociation constant Ka:
Ka = ([H+] + [OH_]) /[H2O](Note that the equilibrium constant is actually a ratio of the activities of the reactants, and activities are equal to concentrations only in solutions that are ideal , i.e. which obey Raoult's Law. In practice, provided that we are dealing with dilute aqueous solutions, we assume that the concentrations of the solutes are the same as their activities because the former are easier to determine than the latter.)
At 25oC the Ka = 1.8 x 10_16 : there is so little dissociation that it is reasonable to say that [H2O] is overwhelming and constant.
We can re_arrange the above equation and introduce a new term Kw the ion product of water:
Kw = Ka[H2O] = [H+] x [OH_] = 10_14 mol dm-3 at 25oCNow in pure water since [H+] must equal [OH_] and multiplied together they = 10_14mol dm-3, therefore [H+] = [OH_] = 10_7mol dm-3.
10_7 x 10_7 = 10_7+(_7) = 10_14To demonstrate the manipulation of indices and logs10:
| 10_7 x 10_7 = 10_14 | _7 + (_7) = _14 | |
| therefore | 10_7 = 10_14 / 10_7 | _7 = _14 _(_7) |
| therefore | 10_7 = 10_14 _(_7) | _7 = _14 _(_7) |
| therefore | 10_7 = 10_14 +7 | _7 = _14 +7 |
Sørensen had the idea of eliminating the minus signs from the log10 of [H+] and invented the pH scale.
The view that Sørensen's idea was misguided has been expressed.
Now in pure water
[H+] = [OH_] = 10_7 mol dm-3If we increase the [H+] of an aqueous solution 100_fold, from 10_7 mol dm-3 to 10_5 mol dm-3, the pH value changes from 7 to 5.
So
the more acid the solution, the lower the pH value;conversely
the pH value rises as the solution becomes more alkaline.The pK of such a group is defined as the pH at which the group is exactly 50% protonated, and is named by analogy to pH: it is -log10K (K = equilibrium constant).
If we now repeat the earlier assumptions about the constancy and overwhelming concentration
of H2O, and the equivalence of H3O+ and H+, we can write:
Ka = [H+][A_]/[HA]This can be written:
[H+] = Ka x [HA] / [A_]Note that since _ log10a / b = + log10b / a, it could be written:
pH = pKa + log10([A_] / [HA])Both are called the Henderson_Hasselbalch Equation: beware of the last term in the equation since many mistakes are made with the sign and decision of which concentration is to be divided by the other.
Consider the effect of putting pH = pKa
Therefore
pKa = pKa _ log10 ([HA] / [A_])Therefore
0 = _ log10([HA] / [A_])This means that the acid is exactly half dissociated.
Consider the effect of putting pH = pKa + 1. This involves a ten_fold change in [H+]:
[H+] is now ten times less than the [H+] at 50% dissociation.
pKa + 1 = pKa _ log10([HA] / [A_])Therefore
1 = _ log10([HA] / [A_])Since antilog 1 is 10, then (remembering the negative sign!)
10 = [A_] / [HA]Therefore:
10[HA] = [A_]This means that if we had eleven molecules of acid, only one would be protonated at that [H+], and ten would be unprotonated.
When pH = pK of the carboxyl group at 2.34, protons will have dissociated from half of the alanine carboxyls present and the net charge will be + 0.5, since the amino group will still be fully protonated and thus carry a positive charge but the unprotonated half of the carboxyl groups present will carry a negative charge.
Further reducing the [H+] of the solution, by adding NaOH for example, will drag the rest of the protons from the alanine carboxyls present and the net charge will be zero. The pK of the amino group of alanine is 9.69 so that when the pH of the solution reaches this value, the net charge will be _0.5.